
P ext and not the pressure of the gas that is used in evaluating the pressure volume work done. It is the external pressure against gas expands i.e.


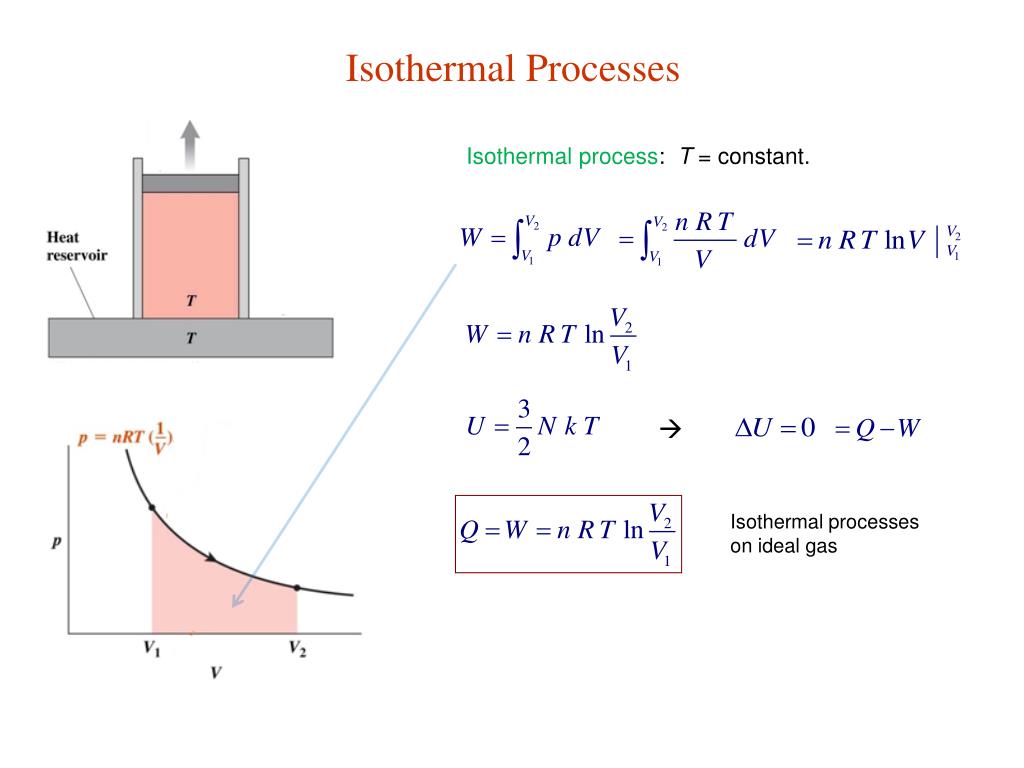
This is an expression for pressure volume work done in terms of pressure and volume in the isothermal expansion of an ideal gas against constant external pressure. Hence the work done by the system is negative. In this process, the system loses energy to the surroundings. Due to expansion, the piston moves upward by a distance ‘d’. Let the gas expand from volume V 1 to V 2 against constant external pressure P ext which is exerted on the piston. Let ‘A’ be the area of the cross-section of the cylinder. $$P_$$This equation acknowledges that, as a result of the viscous property of the gas, the kinetic energy of the piston has been damped out by the time the system reaches its final state.Consider an ideal gas having definite mass (say n moles) be enclosed in a cylinder fitted with weightless, frictionless, tightly fitted, movable piston. In the "vertical piston" example, if one does a force balance on the piston during the process, one obtains: But the ideal gas law (or any other equation of state for that matter), cannot be used to determine the pressure (or more precisely, the compressive stress) in an irreversible process.Īt the interface between the gas and its surroundings (say the inside face of a piston) where the gas is doing work, by Newton's 3rd law, the pressure of the gas matches the "external pressure," and, if this external pressure is known, the amount of work that the gas does on its surroundings can still be determined. In a reversible process, where the rate of change of volume is negligible, the ideal gas law can be used. This is because a gas has a viscous property which acts to dissipate mechanical energy and convert it to internal energy. In a rapid irreversible expansion or compression, the pressure depends not only on the volume (and temperature) but also on the rate of change of volume. The ideal gas law (or other equation of state) is valid only when a gas is in thermodynamic equilibrium. How can you say that it is wasted when the work actually used to increase the kinetic energy of the load as a whole? We always say when p= p ext during expansion, the work done by the system is maximum, otherwise 'work wasted'. With both gases doing the same amount of work, two loads gain the same gravitational potential energy, but one of the loads gains greater kinetic energy, means that one of the loads actually gain greater amount of total energy.
This would mean that, that load actually has higher kinetic energy. However, logically, that piston with higher pressure should move faster and so does the load on it.This is because the load moved up by same vertical distance and the changes in volume of gases are the same for two piston. When both gases expand by the same amount, the work done by both gases, based on the formula d w= - p extd V and Atkins' reasoning, are the same.Envisage the external pressure being a load resting on the piston.Imagine I have two pistons, two filled with different amount of gases at same volume and so different pressure.Now if I use this reasoning on expansion, Is diminished by an amount determined by the weight that is lowered,Īnd it is this energy that is transferred into the system.' 'when a gas is compressed, the ability of the surroundings to do work (b) The explanation given by Atkins' Physical Chemistry (same page) is: Based on Atkins' Physical Chemistry Ninth Edition page 50, we use p ext even for compression. (a) In the case of compression, the opposing force is obviously caused by p but not p ext. Using this reasoning, I cannot explain my following thought processes:

Many of the texts and related questions on this site I encountered give a same general idea: because work done= opposing force x displacement. For irreversible expansion and compression, why is d w= - p extd V but not d w= - pd V ?


 0 kommentar(er)
0 kommentar(er)
